NEOCLASSICAL PHYSICS - CORPUSCULAR QUANTUM MECHANICS
1. ABOUT UNIVERSAL ENERGY of REPULSING
Let's untwist a small weight on cord. Thus the small weight has potential energy of attraction to center of rotation, but let's doubt that the small weight, moving on a circumference, has kinetic energy, and we shall consider of its potential repulsing. It seemed, that this problem of clean convention and has not principled importance, you see accommodating mathematics is capable in appropriate way to formalize and this idea. However in further we shall see, that it not so, that this idea coincides judgement of the Creator on this point. The problem about potential and kinetic energy of a body specially was not studied, since the orthodox science represents apparent always:
- The kinetic energy of a body is a measure of its mechanical motion and is measured by that work, which one can be made by this body at its braking before complete stop.
- The potential energy calls energy dependent only on a positional relationship of interacting mass points or bodies. (B.M. Yavorsky, A.A. Detlaf, "The reference Book on physics for the engineers and students of high schools"," Science ", М., 1964, page 58,59).
J. Orir in the book: "Popular physics" (page 132-134) gives following definitions kinetic and potential energy of bodies.
Half of product of a body mass on a square of its speed is called as kinetic energy of this body. All work, made above a body, on change of value of its speed is exhibited as kinetic energy of a body.
The body
acquires potential energy every time, when it is affected by force Fc
(called conservative), dependent only from its position, but not dependent from
paths. The most common definition of potential energy is given by expression: 
, where - 
angle between a direction Fc
and movement 
s.
At motion on a circumference the body fixed concerning center of rotation, therefore has only potential energy. By releasing a cord, we shall see a small weight, leaving from center, i.e. certain it other condition. Besides releasing a small weight on miscellaneous spacing interval from center of rotation (thus a moment of momentum), we shall be convinced, that the closer to center the small weight is gyrated, the stronger is repulsed from it. The mathematical design of energy of moving on orbit gravitational or diverse charge, as certain kind of potential energy of repulsing (numerically equal mV2/2) does not introduce ados.
In connection with numerous
questions of the readers on generalpurpose potential energy of repulsing, I
shall result in here plus articulatings. The official formulations kinetic and
potential energy are unsufficiently correct. If in a system of interacting
bodies spacing interval between them does not vary, the system has potential
energy. If the body is gone uniformly and rectilinearly, it has kinetic energy.
In all remaining cases a mixed condition at which one the kinetic energy passes
in potential or on the contrary or they are present simultaneously and
separately (the bead is wheels without friction on a horizontal surface). As is
known, the force is of derivative energy on spacing interval. For example,
uniformly driving body has kinetic energy 
. As in
this case V = const, 
, i.e.
resultant all forces is peer to zero point. At motion of a body on a
circumference generalpurpose potential energy of repulsing 
. To find a generalpurpose repulsive force (centrifugal)
before to differentiate WP on radius it is necessary to apply
a law of conservation of angular momentum of a body 
.
If m does not vary, pursuant to this law Vr =
=const. Then 
. Now it is possible to find centrifugal force 
. The angular momentum of a body, driving on a
circumference, can be changed arbitrary, therefore we shall finally have 
. If the connection of a body with center of rotation will
be interrupted, the potential energy of generalpurpose repulsing is transformed
into kinetic energy of a body (spacing interval between bodies, which one
interacted, has become to vary, and the body, leaving from center, is gone
uniformly and rectilinearly).
It is necessary again to return
to this subject with plus explanations. For the readers the absence of kinetic
energy for a body driving on a circumference is not stacked in any way in a
head. We so have got used to consider, that the driving body can somebody
knock. And time can knock - means has kinetic energy. For a determinancy, we
shall consider behavior of a Earth satellite. It appears, that in a direction
perpendicular orbit a satellite anybody to knock can not, therefore has not
kinetic energy in this direction. If it will knock somebody in a direction of
orbital motion or on it, for example, meteorite will knock in that a direction,
the part of potential energy of generalpurpose repulsing will turn to kinetic
energy in a direction perpendicular orbit and the satellite will take in
outcome other orbit with new value of potential energy of generalpurpose
repulsing and zero kinetic energy before following possible impact. Let's
suspect, that in it the meteorite with counter motion has got. In this moment
was destroied the balance between attractive force to the Earth and centrifugal
force. From this equilibrium the expression for the maiden solar escape
velocity on steady orbit is easily received: 
(a).
At the moment of shock the equality (a) was turn intoed an inequality: 
(b). The attractive force to the Earth becomes more
centrifugal force, therefore to restore the status quo it is necessary to
reduce radius of orbit up to a new balance of forces: 
, that is equivalent to increase of potential energy of
generalpurpose repulsing (r1 < r). Thus potential
energy of generalpurpose repulsing receives zero value only on perpetuity and
is augmented at nearing orbit to a central body. In view of a principle of
conservation of moment of momentum the potential energy of generalpurpose
repulsing is inversely proportional to a square of spacing interval up to a
central body, and the energy of a gravitational attraction is inversely
proportional to spacing interval up to a central body in the maiden degree. As
a result of addition, the course of change of potential energy depending on
spacing interval up to a central body will be had a potential well about
existence by which one by an official astronomy does not know. In outcome for
it remains by a riddle, why orbits of planets have an observed osition, instead
of any another.
One more
evidence of absence of kinetic energy for a body driving on a circumference,
consists in following. Is ideate to itself, that in weightlessness a body of
mass m we have attached to a fixed axis of rotation on a lengthy thread,
stretched slightly thread and have pushed a body in a direction a perpendicular
thread with near-zero speed. Let's consider, that in the initial moment our
system had zero kinetic and potential energy because of minor speed of an
initial rotation about an axis. Step-by-step thread is wound on rotation axis,
its length decreases, and the peripheral speed of motion of mass is augmented.
What we shall watch at decreasing a radius of gyration, for example, in 2
times? Apparently, that the peripheral speed of rotated mass will become
considerable and ad lib large at sufficient initial length of a thread.
Apparently, that the energy of our system was increased up to considerable
value. Now it is necessary to find out, whence it has appeared and that it
energy. Perpetuum mobile otherwise is easy to create, which one catches flying by
subjects, untwists them and rejects with large kinetic energy. Rotated mass is
under operating of two forces - force of pull of a thread and centrifugal
effort. These forces are not balanced, since force of pull of a thread all time
it is a little more centrifugal effort at the expense of that mass is
step-by-step displaced to center of rotation. Thus, the work is effectuated by
thread, overcoming centrifugal effort. Apparently, that the value of this work
will be peer 
, where V - reached peripheral
speed of mass. The obtained energy in any way cannot be connected with kinetic
energy of mass since in a direction to perpendicular motion any force work does
not commit, and in a direction of peripheral speed any force does not act. The
sanction of this paradox is, that at approach with rotation axis the potential
energy of generalpurpose repulsing is augmented, and to what speed there is
this approach has not value, that is characteristic just for potential energy.
The situation is similar to that, at which one the crane lifts shipment,
winding on a rope on a barrel.
Thus, I have answered problems the А. Poincare concerning potential energy, which one till now remained without the answer (G.M. Golin, C.R. Filonovich. Classics of physical science. М., 1989, page 492): «That to materialize energy it is necessary to localize, it; concerning kinetic energy it is simple, but not so the business with energy potential is. Where to localize potential energy called by attraction of two celestial bodies? In one of two? In both? In an interspace?».
One more problem, on which one is necessary to be stopped by consideration of motion of a body on a circumference, is a problem of existence of a centripetal acceleration not as mathematical abstraction, and as physical reality.
The man on equator is under operating of «centripetal acceleration», which one is piled with acceleration of gravity, therefore should weigh more, than on a pole. However actually all is on the contrary: the centrifugal force partially compensates force of weight. Therefore fictitious not centrifugal force is (as assert orthodoxes) and «centripetal acceleration».
The
acceleration calls a vector quantity w, describing a rate of change of
speed of a driving point and equal first derivative from speed on time: 
. The acceleration vector lies in an adjoining plane passing
through a main normal and tangent to a trajectory, and is directed to the side
of a concavity of a trajectory (B.M. Yavorsky, A.A. Detlaf "The reference
Book on physics for the engineers and students of high schools"," Science
", М., 1964, page 20).
The concept of a centripetal acceleration is entered by a different image. We shall take advantage of the book: J. B. Marion “Physics and the Physical Universe”, “World”, M., 1975, page 126. Let's consider equable motion of a body on a circumference.
As the velocity vectors V1 and V2 are perpendicular to radiuses of a circle r, a triangle,
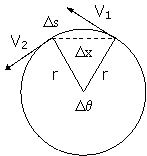
formed in two radiuses and chord x, is alike to a triangle
formed V1, V2 and V. both triangles isosceles,
and the angles between the equal legs for them are identical and are peer . Thus, numerical value of an
alteration speed: 
. The acceleration is determined by expression: 
.
Substituting
value V
and taking out for the sign of a limit of constants, we shall receive: 
. At decreasing t up to infinitesimal values the chord x becomes to an equal arc s, and the limit of ratio s/t is peer to peripheral speed V.
Therefore: 
. It is easy to see, that the
acceleration vector conterminous on a direction with vector V, will be directed to center of a
circumference if to esteem infinitesimal period.
If to consider,
that the body, driving on a circumference, has potential energy of repulsing,
on a problem on existence of a centripetal acceleration we at once receive uniquely
negative answer. When the official science states, that acceleration - the
vector quantity having a direction, conterminous with a direction of change of
velocity vector in course of time, and results the applicable formula and
scheme of definition of a difference of vectors, it puts a cart ahead of the
horse in the sense that causes physics adequately to mirror to mathematics,
rather the reverse. From the physical point of view the main criterion of even
movement of a body on a circumference extreme is simple: the peripheral speed
of its motion remains to a constant V = const. Let's try to
translate this outgiving to the language of mathematics. Apparently, that, in
this case, it is necessary to consider speed not vectorial, and scalar value.
Otherwise translation will become unidentical and will gain opposite physical
sense V
const,
since identical the vectors with an equal module and only parallel each other
are considered. As mathematics can not give more that in it is originally
enclosed, it and gives us acceleration, which one does not exist. It is one of
examples that we were not learned yet correctly to translate into the language
of mathematics even simple physical notions, it concerns and to return
translation. For the incorrigible fans to juggle with vectors, it is possible
in general to refuse speed with arrow and to exchange by its ratio of length of
orbit to a period of rotation. Such ratio at all desire is impossible to
present as vector, since numerator and denominator of its scalar value.
There is a simple experimental capability to reduce to zero point attractive force to center of rotation and at the same time to leave without change a repulsive force from center. For this purpose it is necessary to be inside the capsule, the walls are hardly connected which one to center of rotation. Inside this capsule we shall completely legibly fix change of a repulsive force depending on a radius of gyration at the same mechanical moment of system not only simple physical experiments, but also own feels. Let's try to lower a bead on a floor of this rotated capsule. It trundles with acceleration instituted second Newton's law, under operating of force of universal repulsing, but not to rotation axis, and in the counter side, also will stay only then, when the force of universal repulsing which is operational on a bead, will be balance by reaction of the capsule wall, which one also acts on a bead.
One more weighty argument that acceleration at motion on a circumference misses consists in following. The energy conservation law regulates radiation of photons only at negative acceleration of electric charge (bremsstrahlung), at positive acceleration the energy is occluded from an environment and the radiation is impossible. "Acceleration", bound only with a veering of velocity vector has not neither positive, nor negative sign. On the language of mathematics it means zero point, and on the language of physics - absence of acceleration. Synchrotron emission, which one result, ostensibly, direct evidence of radiation at even movement of electric charge on a circumference, will be reviewed in the theory of atom (see also chapter 11.5); it has completely other nature.
In a considered case a centripetal force (it is better to tell - attractive force) is perpendicular to velocity vector, therefore, work does not commit also acceleration does not cause. On this basis the introducing of concept of a centripetal acceleration has inflicted an apparent harm to physics (especially to physics of a microcosmos), though and it is understandable, as aspiration "to keep" the second Newton's law. Below we shall see that in case of motion of a body on a circumference the second Newton's law is fair, but for other reasons.
The even movement of a body on a circumference is full analogy of even movement of a bead on a horizontal surface. Thus the attractive force of a bead to the Earth applied on a bead, is equilibrated by forces of reaction of an abutment surface also applied on a bead, therefore bead rest in a direction of operating of these forces (as is in a potential well). Thus nothing hinders it uniformly to move in a perpendicular direction, not committing work. Precisely that takes place and at motion of a body on a circumference, where the attractive force can be of any genesis, and the repulsive force from center of rotation is universal for any bodies. These two forces are counterbalanced, and the motion in a direction, perpendicular operating of forces, takes place without accomplishment work.
 |
Negating existence of a centripetal acceleration, we simultaneously negate also first Newton's law for motion of a body, putting in completely equivalent conditions even movement of a body on a circumference and even rectilinear motion. Running forward, it is possible to tell, that any free bodies, starting from microparticles and finishing space objects simultaneously will realizes these two rights in an equal measure, moving on a screw line with equal translational and tangential velocity. Further we shall see, that for comprehension of motion of microparticles and space objects it is necessary to sacrifice not only first, but also third Newton's law and this victim appears justified. After such statement the author is sure, that the adherents of doctrines in science is abandoned a reader's auditorium, therefore addresses to stay with the request to be patience, which one will be compensated.
For the greater clearness of this problem we shall consider, at first blush, indisputable case, in which one the driving electric charge does not beam photons - even and rectilinear motion it (figure 1.1).
The electric charge of mass m uniformly moves
on a straight line АВ from perpetuity A
in perpetuity В. We shall take an arbitrary
point C with which one a charge does not interact in any way. Let's
consider motion of a charge comparatively this point. In a point 1
shortest distance from С up to АВ a moment of momentum of a charge comparatively C: 
. For any
arbitrary point 2 on a straight line АВ it is possible to find a
projection V1 on tangent to a circumference of radius r2,
which one we shall designate V2. From a similarity of two
triangles is discovered, that 
.
All reasoning will not change, if the body m was gyrated around of a point C, and in a point 1 connection has broken. Thus, at even and rectilinear motion of a body its angular momentum of concerning any point of space remains to a constant and explains inertia of bodies. The inertia is a demonstration of a law of conservation of angular momentum of a body. Any attempts to change speed of a body or direction of this speed are connected to energy consumption on change of angular momentum of a body.
This outcome is possible to interpret, as rotation of a charge of mass m around of a point C in full conformity with a principle of conservation of moment of momentum S. Thus the charge "is attracted" to a point C, reducing a radius of gyration and, accordingly, augment speed rotations, and then "is repulsed" from it, augmenting a radius of gyration and decreasing speed rotations. The truth, in all this process at motion of a charge from A in B is committed of all half-revolution around of a point C, but the heart of the matter from it does not vary. The electric charge does not beam energy, not only moving on a fixed circular orbit, but also on orbit of any form, under condition of preservation of a total energy, or if it is in a potential well (see theory of atom). Thus it can participate and in other motions, thus a condition. Who disagrees with this conclusion, insists that the capability of radiation depends on selection of a reference system, that obvious nonsense. And how to be with conclusions of an electrodynamics, you ask, which one uniquely indicate that the charge, driving on a circumference, should lose energy through electromagnetic radiation? Here official physics will use for a fraud of the reader a following method. The theory of radiation of an electric dipole applies to motion of electric charge on a circumference. If one spectator sees along a plane of rotation oscillating electric charge and this motion could be compared to a dipole, let's put the second spectator on the angle 900 comparatively first spectator. One spectator will assert that the radiation should be outspread to it, and to the partner of radiation is not present. Precisely same conclusion will be made also by the second spectator. The radiation simultaneously is, and it is not present, that indicates incompetence comparison of motion electric charge on a circumference with an electric dipole. The comparison of orbital motion of an electron with an oscillating dipole is erratic also that for one vibration period of a dipole the positive acceleration doubly for period is replaced by negative acceleration, and centripetal (new physics negates its existence) acceleration of an electron permanently. Nevertheless, official physics widely will use not adequate comparisons. In conditions monopolism on true there is nobody to indicate errors. Further we shall see that we is called as an electromagnetic wave has completely other physical nature, and the Maxwell equations have appeared on that to a simple reason, that for him under an arm it was not simple more anything applicable, to explanation self-propagation of light in space.
The denying of existence of a centripetal acceleration automatically removes a problem on energy loss by an electron driving around of a nucleus, as it is gone without acceleration. The quantum mechanics recognizes existence of an electron angular momentum in atom, therefore, should recognize the fact of motion of a S-electron around of a nucleus and to explain stability of atom. In this respect first postulate of the Bohr is more correct, though it and does not explain a reason absence of radiation.
The first
postulate of the Bohr: the electrons can move in atom only on definite orbits,
being on which one, they, despite of presence for them of acceleration
(is selected by me - V.K.), do not beam. These orbits correspond to stationary
states of electrons in atom and are determined by a condition: 
, where rn - radius n-th of orbit, me∙vn∙rn
- moment of momentum of an electron on this orbit, h - constant of the
Planck, n - integer (n>0). B.M. Yavorsky, A.A. Detlaf
"The reference Book on physics for the engineers and students of high
schools"," Science ", М., 1964, page 670-671.
From further it will be visible, that mV2/2 is universal potential energy of repulsing of any interacting systems, starting from galaxies and finishing the constituents of "elementary" particles. The repulsing of like electric charges and like magnetic poles, as we shall see, has not crucial importance in comparison with mV2/2 specially for systems affected in this work.
To open this universal potential energy of repulsing in an obvious kind depending on radius of orbit of a driving gravitational charge (some mass) or electric charge (too possessing simultaneously and gravitational charge), that is completely necessary for applying the mathematical apparatus, we shall decide a of equations set:
(1.1),
(1.2),
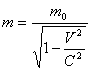(1.3).
 |
By the formula (1.3) we shall take advantage for the lack of anything best for connection of mass of a body with speed of its motion (see chapter about "elementary" particles, where the physical sense of this formula is uncovered and chapter 5.2).
The solution of equations set:
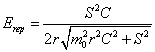(1.4).
At r large (this case overlaps range of the
sizes from radius of atom up to radiuses of galaxies and corresponds to absence
of noticeable relativistic increase of mass, 
considerably
exceeds S2), from (1.4):
(1.5).
At r small (this case concerns to
"elementary" particles and their internal parts and corresponds to
considerable relativistic increase of mass, when 
, is
significant less S2), from (1.4):
(1.6).
Substituting 
in (1.5) and 
in (1.6),
it is easy to be convinced that we shall receive in first case 
, and in second
.
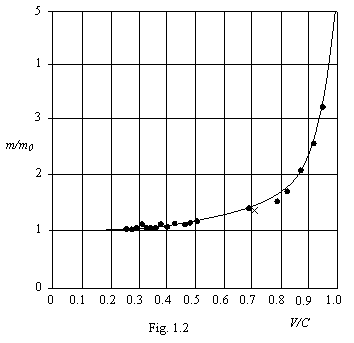
Experimental (1901-1909) relation of electronic mass to speed of its motion (point). The curve corresponds to the formula (1.3) is shown on a figure 1.2.
The graph is borrowed from the book: J.B. Marion "Physics and physical world", "World", М., 1975, page 30. The dagger on the graph is put by the author and the sense it will be uncovered at the description of an electron (see also chapter 5.2).
The so universal potential energy of attraction, apparently, does not exist, for example, the attraction of space objects is determined by gravitational forces, electrons to a nucleus of atom - electrostatic, and nucleones in a nucleus - nuclear. All the same, running forward, it is possible to tell, that all parameters of space systems, atoms and "elementary" particles, at the end, are determined by universal potential energy of attraction, the kind of a function by which one depends on concrete circumstances, and which one conditionally we shall call as gravidynamic attraction. The gravitational charges, rotated about the axis and migrating in space are necessary for its development. As all in the Nature is anyhow gyrated, and even rectilinear motion only abstraction, which one is not present conformity in a substantial world (that with all evidence will be shown below), the developments of gravidynamic interplay are easy for watching both in space scales, and in a microcosmos, and starting from nuclei of atoms and finishing the constituents of "elementary" particles, the gravidynamic attraction is exhibited practically in the pure state. Here it is necessary at once to be stipulated, that in the book there are no declarative applications. Early or late validity of each expression will be show. For example, will be shown, that all free bodies moving on a screw line, and the first Newton's law is fair to an axis of this line, but not to the body.
Comments of the author to chapter 1:
1. One more evidence of existence of a repulsing potential field at motion on a circumference.
Here I shall result in mathematical reasons for the benefit of universal potential energy of repulsing at motion of a body on a circumference.
As though we did not trice a
body to center of a circumference (sharply or step-by-step) or did not release,
that remove from center, the common energy will be peer to zero point, if we
shall return to initial point on that spacing interval from center, (in any
point of a circumference on this spacing interval we shall get without energy
consumption). Let's presume, in initial position to a body m on a circumference
with peripheral speed V0 and having «kinetic» energy E we have
imparted plus energy W, then, apparently, that:
(1), where V - new (current) peripheral
speed. From (1): 
(2).
Let's record angular momentum of a body on a circumference: 
(3), where R - radius of gyration.
Let's erect (3) in a square and we shall record a square of speed: 
(4). Let's substitute (4)
in (2):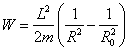 
(5).
Let's substitute in (5) expressions of angular momentum and is reform: 
(6). The expression (6)
demonstrates, that the energy, which is possible to receive (or to expend) at
change of a radius of gyration is inversely proportional to a square of spacing
interval from center of rotation and in this sense the potential field of
universal repulsing by nothing differs from an electrostatic or gravitational
potential field, only potential field of repulsing more abruptly changes with
spacing interval from center (not E ~ 1/R, and E ~ 1/R2). It also
determines existence of a potential well at electrostatic and gravitational
interaction. Without potential energy of repulsing the existence of a potential
well of interplay is impossible.
About kinetic energy of a body moved on a circumference is possible to reason only for a body, is hard bound with center of rotation. In this case any change of kinetic energy of a body does not result in change of a radius of gyration, i.e. the law of conservation of angular momentum in this case does not work also potential energy of repulsing does not vary.
1.1. At motion of bodies on a circumference accelerations miss
 |
On a figure 1.1.1 the disk 1 radius R is shown. The rod 2 is hardly connected to the disk on which one freely some mass m can to slip. Let's begin to twist the disk with constant angular velocity w. Apparently, that position m in center of the disk is unstable also this mass will begin to slip on a rod 2 to peripherals of the disk under operating of centrifugal force (not centripetal force, which one here in general is not present). As, in this case, simultaneously varies both radius of gyration of mass m and its linear peripheral velocity:
V=
×r
(1.1.1),
that, apparently, that formula of centrifugal force as:
(1.1.2)
to apply it is impossible. Let's substitute (1.1.1) in (1.1.2):
(1.1.3).
From the second Newton's law is apparent, that the centrifugal acceleration for considered mass will be:
(1.1.4),
which one formally coincides taking into account (1.1.1) with expression for «centripetal» acceleration of official physics:
(1.1.5).
As the centrifugal force pursuant to (1.1.3) linearly increases with radius, its mean active value will be equal to half maximum. Then work accomplished by this force on path r:
(1.1.6).
The kinetic energy of a material point of mass m is determined by the same expression:
, where 
-
moment of inertia of mass m (1.1.7).
If on the end of a rod to put a bearing, the centrifugal force will be balanced by reacting of this bearing and in a direction of operating of these forces movement and any acceleration miss. In this case considered mass has kinetic energy pursuant to the formula (1.1.7). To make an analysis demonstrates, that speculations of official physics around of «centripetal» acceleration are erratic. In a considered problem there is only centrifugal acceleration and that only so long as we permit to mass freely to move along radius. At motion of a body on a circumference with fixed radius of any accelerations is not present.
I want to remind to the adherents of vectorial world outlook, that the square of vector is a scalar not having directions. In the formula (1.1.5) vectors in general is not present, even if peripheral velocity to consider as vector. On this basis the radiation of electric charge driving on a circumference is impossible since it has not acceleration any of a direction. Therefore scientists have made in due time large error by rejecting the theory of atom of the Bohr.
In chapter 1 is shown, that at even rectilinear motion the angular momentum of a body with respect any point of space does not vary. Direct consequence of this conclusion is the definition of inertia of a body, as manifestation of a fundamental law of conservation of angular momentum of a body. While the angular momentum of a body does not vary, inertia we is not observed. As soon as we begin to accelerate or to slow down motion or to change its direction, then there is an inertia. Any change of angular momentum is connected to operating of force and it, in its turn, is determined by speed of change of an impulse of a body pursuant to the second Newton's law:
(1.2.1)
If force F to decompose on three axes of coordinates, (1.2.1) it is possible to copy in the scalar form of speed of change of components of an impulse on these axes. This fact indicates independence of motions under operating of any component summary force. Thus, pursuant to the second Newton's law, the slower angular momentum of a body changes, the the smaller force needs to be applied to this body.
 |
The versions of change of angular momentum for rectilinear motion of a body are shown on figures 1.2.1 and 1.2.2.
On a figure 1.2.1 as a result of operating on a body of some force on a segment AB, having angular momentum mV1h concerning an arbitrary point C, its angular momentum has changed and has become equal mV2h. On a figure 1.2.2 as a result of operating on a body of some force on a segment AB having a moment mV1h1 concerning an arbitrary point C, its angular momentum has changed and has become equal mV1h2.
Here it is necessary to recollect the third Newton's law: the operating is equal to counteraction, and the counterforce is directed to opposite operational force. If formally to apply this law, it is possible to reach to in principle error conclusions. For example, we act on a body with the purpose to change its speed or current of traffic, but, meeting equal and the opposite directional counteraction, we can not change parameters of motion of this body. The picture of interplay of bodies becomes clear in experiment, when the weight put on a cushion. In this experiment the weight, despite of a contact with a surface of a cushion, in the beginning is gone accelerated under operating of attractive force to the Earth. In this moment the counterforce of a cushion smoothly accrues from zero point up to some value, equal difference of weight of the weight and force of inertia it, since the force of inertia of the weight is directed opposite to weight. The weight is gone to some instant already uniformly and its force of inertia disappears. The resistance force of a cushion prolongs to be augmented and the weight is gone already in a slowed-up way, the force of inertia arises again and now it is directed on a direction of the operational force of weight. Therefore the weight sags a surface of a cushion is more, than in a case of indefinitely sluggish lowering of the weight. There comes the moment, when the weight is gone back. After several oscillations or aperiodic damping them, is struck the balance, at which one of weight of a body applied to the weight is balanced counteraction of a cushion also applied on the weight. Therefore the weight is in a state of rest. For brevity, we here not consider interplay of a cushion with a bed, bed with a floor, floor with the Earth, Earth with the Sun etc. Apparently, as all bodies consist of atoms, is ideally of rigid bodies in the nature there is no also interplay by their direct contact to the similarly reviewed interplay of the weight with a cushion. It is possible to make of described experiment such conclusion: the third Newton's law is fair only for equilibrium state of a system, which one can be both static, and dynamic (revolution). Three interdependent forces participate in interplay of bodies: force of operating, force of inertia and counterforce. The operational force is independent of time, and force of inertia and counterforce depend on time of interplay, and the force of inertia instantaneously reacts to change of angular momentum of a body.
Let's consider now case of interplay at roundabout of a body. In this book the numerous evidences are adduced that all bodies in the Nature move on a screw line. The force of inertia is present at a tangential direction of this motion permanently as centrifugal effort and is conditioned by equalling of this force and force of gravidynamic attraction to an axis of a screw trajectory of a body. Therefore reasoning tangent figures 1.2.1 and 1.2.2 are completely fair to an axis of a screw trajectory of bodies (to a translational motion of a body). On a figure 1.2.3 is shown interplay of a body 1 with a central body 2 (it there can be a man who is winding on spool a thread, linking him with a body 1 or the atom nucleus on which one «drops» an electron).
 |
The equilibrium state here is reached not at once, is similar to dip of the weight on a cushion and consequently allows explicitly to consider interplay.
In not relativistic area mass of a body 1 does not vary (m0), the angular momentum it depends only on product
Vr
= = const (1.2.2),
therefore angular momentum:
m0Vr
= m0 (1.2.3).
Let's multiply both parts (1.2.3) on V/2r, then with the registration (1.2.2) we shall receive:
Ek
= m02/2r2
(1.2.4),
where Ek - kinetic energy of a body. Actually (1.2.4) not kinetic energy of a rotated body, and potential energy of generalpurpose repulsing, numerically equal mV2/2 (chapter 1).
Differentiating (1.2.4) on radius with the subsequent substitution (1.2.2), we shall discover:
Fcf = -m0V2/r (1.2.5),
where Fcf - centrifugal effort (force of inertia or force of generalpurpose repulsing in this case).
In relativistic area the running speed is peer to speed of light C, therefore angular momentum depends only on product
mr
=
= const (1.2.6),
where m - relativistic mass of a body, therefore angular momentum:
mCr
= C
(1.2.7).
Multiplying both parts (1.2.7) on C/2r with the registration (1.2.6) and by repeating the manipulations similar above-stated for not relativistic case, we shall receive relativistic force of generalpurpose repulsing:
Fcf = -mC2/2r (1.2.8).
In considered interplay of bodies the operational force on the part of a body 2 on a body 1 can be of any genesis and to change under any law depending on spacing interval between bodies. Thus operational on a body 1 force is more than an arising counteractive force by which one, in this case, is the centrifugal effort of inertia so long as they will not become equal at the expense of increase of force of inertia of a body 1. Thus the body 1 will move on a fixed circular trajectory indicated on a figure by 1.2.3 dotted line. In this condition the attractive force acts on a body 1 on the part of a body 2 and the force of generalpurpose repulsing acts equal to it on the same body. Therefore body 1 rest in a direction of operating of these forces, but is gone in a perpendicular direction without undertaking work to a similarly rolling sphere on a horizontal plane. In under consideration a case the counterforce coincides force of inertia and is peer to the operational force only in equilibrium state.